(중간저장) |
|||
| 4번째 줄: | 4번째 줄: | ||
[[파일:Circle radians.gif|섬네일|300px]] | [[파일:Circle radians.gif|섬네일|300px]] | ||
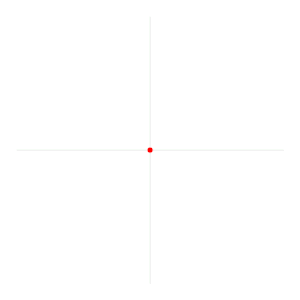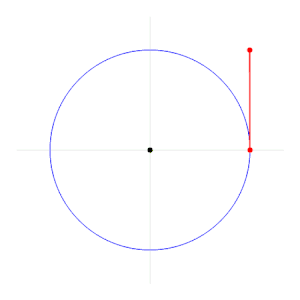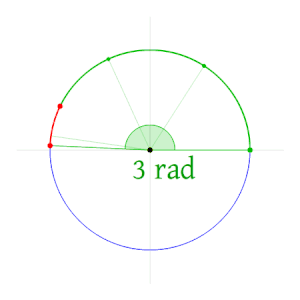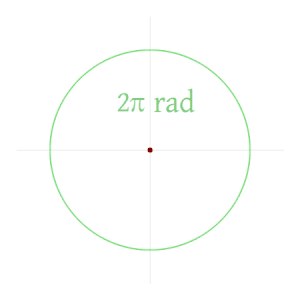
[[부채꼴]]의 반지름과 호의 길이가 같을 때 중심각의 크기를 1'''라디안(radian)'''이라고 하고, <math>1\operatorname{rad}</math>로 쓴다. 보통 <math>\operatorname{rad}</math>는 생략한다. 예를 들어, <math>\sin (1\operatorname{rad})</math>은 <math>\sin 1</math>로 간단하게 표기한다. | [[부채꼴]]의 반지름과 호의 길이가 같을 때 중심각의 크기를 1'''라디안(radian)'''이라고 하고, <math>1\operatorname{rad}</math>로 쓴다. 보통 <math>\operatorname{rad}</math>는 생략한다. 예를 들어, <math>\sin (1\operatorname{rad})</math>은 <math>\sin 1</math>로 간단하게 표기한다. | ||
== 역사 == | |||
라디안이 사용된 것은 비교적 최근으로 알려져 있다. | |||
== 60분법과의 관계 == | == 60분법과의 관계 == | ||
| 58번째 줄: | 61번째 줄: | ||
상술한 이유로 라디안은 원주율과 합쳐서 각도를 나타낼 때도 있는데 이때 °로 끝나지 않기 때문에 착각을 하기가 쉽다. 상술했듯이 원주율 뒤의 rad를 생략할 때가 많아서서 π/2가 90°의 각도라는 것을 모르고 이게 길이인 줄 알고 잘못 계산하는 경우가 있다. 조심하자. | 상술한 이유로 라디안은 원주율과 합쳐서 각도를 나타낼 때도 있는데 이때 °로 끝나지 않기 때문에 착각을 하기가 쉽다. 상술했듯이 원주율 뒤의 rad를 생략할 때가 많아서서 π/2가 90°의 각도라는 것을 모르고 이게 길이인 줄 알고 잘못 계산하는 경우가 있다. 조심하자. | ||
부채꼴의 반지름의 길이와 호의 길이가 같을 때, 반지름을 <math>r</math>이라 하면 | |||
: <math>2\pi r : 360^\circ = r:\alpha^\circ</math> | |||
에서 | |||
: <math>\alpha^\circ = \frac{180^\circ}{\pi}</math> | |||
이므로 | |||
: <math>1\operatorname{rad}=\frac{180^\circ}{\pi}</math> | : <math>1\operatorname{rad}=\frac{180^\circ}{\pi}</math> | ||
그런데 [[2015 개정 교육과정]]을 따르는 [[미적분Ⅱ]] [[교과서]]를 검토하면 대부분이 일정한 각의 크기 <math>\frac{180^\circ}{\pi}</math>를 <math>1\operatorname{rad}</math>이라 한다<ref>{{저널 인용|저자=최은아, 강향임|연도=2015|월=9|제목=예비교사의 라디안에 대한 이해|저널=학교수학|권=17|호=2|쪽=313-314}}</ref> | 을 얻는다. 그런데 [[2015 개정 교육과정]]을 따르는 [[미적분Ⅱ]] [[교과서]]를 검토하면 대부분이 라디안을 "일정한 각의 크기 <math>\frac{180^\circ}{\pi}</math>를 <math>1\operatorname{rad}</math>이라 한다"라고 정의하고 있는데, 이는 라디안이 새로운 측도가 아니라 단순히 60분법의 변환에 불과하다는 인상을 준다는 단점이 있다.<ref>{{저널 인용|저자=최은아, 강향임|연도=2015|월=9|제목=예비교사의 라디안에 대한 이해|저널=학교수학|권=17|호=2|쪽=313-314}}</ref> | ||
== 호의 길이와 넓이 == | == 호의 길이와 넓이 == | ||
| 89번째 줄: | 97번째 줄: | ||
: <math>\sec\theta=\frac{r}{x}</math> | : <math>\sec\theta=\frac{r}{x}</math> | ||
: <math>\cot\theta=\frac{x}{y}</math> | : <math>\cot\theta=\frac{x}{y}</math> | ||
삼각함수는 실수 집합에서 실수 집합으로 가는 함수가 맞을까? | 삼각함수는 실수 집합에서 실수 집합으로 가는 함수가 맞을까? 래핑을 통한 은유가 제안되기도 한다.<ref>{{저널 인용|저자=Hatice Akkoc|연도=2008|월=10|제목=Pre-service mathematics teachers’ concept images of radian|저널=International Journal of Mathematical Education in Science and Technology|권=39|호=7|쪽=876}}</ref> 실제로 Precalculus 교재 일부는 | ||
포장함수(wrapping function) <math>W(x)</math>를 정의한다.<ref>{{서적 인용|제목=Precalculus: Functions and Graphs|저자=Raymond A. Barnett, Michael R. Ziegler, Karl E. Byleen|url=http://www.mhhe.com/math/precalc/barnettpc5/graphics/barnett05pcfg/ch05/others/bpc5_ch05-01.pdf|확인날짜=2016-04-12|판=5th edition|날짜=2000-07-01|출판사=McGraw-Hill Science/Engineering/Math|ISBN=978-0072368710|장=Chapter 5, Section 1}}</ref> | 포장함수(wrapping function) <math>W(x)</math>를 정의한다.<ref>{{서적 인용|제목=Precalculus: Functions and Graphs|저자=Raymond A. Barnett, Michael R. Ziegler, Karl E. Byleen|url=http://www.mhhe.com/math/precalc/barnettpc5/graphics/barnett05pcfg/ch05/others/bpc5_ch05-01.pdf|확인날짜=2016-04-12|판=5th edition|날짜=2000-07-01|출판사=McGraw-Hill Science/Engineering/Math|ISBN=978-0072368710|장=Chapter 5, Section 1}}</ref> | ||
: <math>W(0)=(1,0)</math> | : <math>W(0)=(1,0)</math> | ||
2016년 4월 13일 (수) 18:42 판
정의
부채꼴의 반지름과 호의 길이가 같을 때 중심각의 크기를 1라디안(radian)이라고 하고, [math]\displaystyle{ 1\operatorname{rad} }[/math]로 쓴다. 보통 [math]\displaystyle{ \operatorname{rad} }[/math]는 생략한다. 예를 들어, [math]\displaystyle{ \sin (1\operatorname{rad}) }[/math]은 [math]\displaystyle{ \sin 1 }[/math]로 간단하게 표기한다.
역사
라디안이 사용된 것은 비교적 최근으로 알려져 있다.
60분법과의 관계
라디안과 60분법의 관계를 알기 위해서는 세 가지를 머릿속에 담고 있어야 한다.
첫번째로, 원주율에 대해서 명심할 사실이 있는데 π×(원의 지름)=(원의 둘레)라는 사실이다. 양변에 똑같이 2를 나눠보면 π×(원의 지름)/2=(원의 둘레)/2가 되므로 π×(원의 반지름)=(반원의 호의 길이)가 된다.
두번째로, 부채꼴에서 중심각의 크기는 그 부채꼴의 호의 길이에 비례한다는 사실이다.
세번째로, 중심각이 1rad인 부채꼴의 호의 길이는 반지름과 같다.이다.
따라서
π×(원의 반지름):π×1rad=(반원의 호의 길이):(반원의 중심각의 크기)
이렇게 되는데 상술했듯 π×(원의 반지름)=(반원의 호의 길이)이므로
(같은 거):π×1rad=(같은 거):(반원의 중심각의 크기)
가 된다. 서로 같은 것에 대한 비례가 같다는 게 무슨 뜻이겠어? 당연히 그것들도 같다는 뜻이다.
결국
π×1rad=(반원의 중심각의 크기)
가 되므로
'πrad=180°'이다.
| 도 | 라디안 |
|---|---|
| 0° | 0 |
| 15° | π/12 |
| 30° | π/6 |
| 45° | π/4 |
| 60° | π/3 |
| 90° | π/2 |
| 180° | π |
| 360° | 2π |
상술한 이유로 라디안은 원주율과 합쳐서 각도를 나타낼 때도 있는데 이때 °로 끝나지 않기 때문에 착각을 하기가 쉽다. 상술했듯이 원주율 뒤의 rad를 생략할 때가 많아서서 π/2가 90°의 각도라는 것을 모르고 이게 길이인 줄 알고 잘못 계산하는 경우가 있다. 조심하자.
부채꼴의 반지름의 길이와 호의 길이가 같을 때, 반지름을 [math]\displaystyle{ r }[/math]이라 하면
- [math]\displaystyle{ 2\pi r : 360^\circ = r:\alpha^\circ }[/math]
에서
- [math]\displaystyle{ \alpha^\circ = \frac{180^\circ}{\pi} }[/math]
이므로
- [math]\displaystyle{ 1\operatorname{rad}=\frac{180^\circ}{\pi} }[/math]
을 얻는다. 그런데 2015 개정 교육과정을 따르는 미적분Ⅱ 교과서를 검토하면 대부분이 라디안을 "일정한 각의 크기 [math]\displaystyle{ \frac{180^\circ}{\pi} }[/math]를 [math]\displaystyle{ 1\operatorname{rad} }[/math]이라 한다"라고 정의하고 있는데, 이는 라디안이 새로운 측도가 아니라 단순히 60분법의 변환에 불과하다는 인상을 준다는 단점이 있다.[1]
호의 길이와 넓이
반지름이 [math]\displaystyle{ r }[/math], 중심각이 [math]\displaystyle{ \theta }[/math]인 부채꼴의 호의 길이를 [math]\displaystyle{ l }[/math], 부채꼴의 넓이를 [math]\displaystyle{ S }[/math]라 하면
- [math]\displaystyle{ l=r\theta }[/math]
- [math]\displaystyle{ S=\frac{1}{2}r^2\theta = \frac{1}{2}rl }[/math]
이다.
Proof 중심각의 크기에 정비례하기 때문에
이고, 따라서 호의 길이는
이다. 마찬가지로 부채꼴의 넓이도 중심각의 크기에 정비례하기 때문에
이고, 따라서
|
여기서 [math]\displaystyle{ l=r\theta }[/math]에 등장하는 [math]\displaystyle{ \theta }[/math]에는 [math]\displaystyle{ \operatorname{rad} }[/math]이 붙어있지 않다. [math]\displaystyle{ \operatorname{rad} }[/math]가 붙어 있다고 가정하고, [math]\displaystyle{ r,l }[/math]에 길이 단위인 미터를 붙이면
- [math]\displaystyle{ l\;(\mathrm{m})=r\theta\;(\mathrm{m\times rad}) }[/math]
으로 단위 차원이 일치하지 않기 때문이다.[2]
포장함수
원 [math]\displaystyle{ x^2+y^2= r^2\;(r\gt 0) }[/math] 위의 임의의 한 점을 [math]\displaystyle{ \mathrm{P}(x,y) }[/math]라 하고, [math]\displaystyle{ \mathrm{A}=(r,0) }[/math]이며, [math]\displaystyle{ \theta=\angle \mathrm{POA} }[/math]라 하면 삼각함수는 다음과 같이 정의된다.
- [math]\displaystyle{ \sin\theta=\frac{y}{r} }[/math]
- [math]\displaystyle{ \cos\theta=\frac{x}{r} }[/math]
- [math]\displaystyle{ \tan\theta=\frac{y}{x} }[/math]
- [math]\displaystyle{ \csc\theta=\frac{r}{y} }[/math]
- [math]\displaystyle{ \sec\theta=\frac{r}{x} }[/math]
- [math]\displaystyle{ \cot\theta=\frac{x}{y} }[/math]
삼각함수는 실수 집합에서 실수 집합으로 가는 함수가 맞을까? 래핑을 통한 은유가 제안되기도 한다.[3] 실제로 Precalculus 교재 일부는 포장함수(wrapping function) [math]\displaystyle{ W(x) }[/math]를 정의한다.[4]
- [math]\displaystyle{ W(0)=(1,0) }[/math]
- [math]\displaystyle{ W(1)=(0,1) }[/math]
- [math]\displaystyle{ W\left(\frac{3}{4}\pi\right)=\left(-\frac{\sqrt{2}}{2}.\frac{\sqrt{2}}{2}\right) }[/math]
[math]\displaystyle{ \cos\theta }[/math]는 [math]\displaystyle{ W(\theta) }[/math]의 [math]\displaystyle{ x }[/math]좌표, [math]\displaystyle{ \sin\theta }[/math]는 [math]\displaystyle{ W(\theta) }[/math]의 [math]\displaystyle{ y }[/math]좌표로 정의한다.[5]
같이 보기
각주
- ↑ 최은아, 강향임 (2015년 9월). 예비교사의 라디안에 대한 이해. 《학교수학》 17 (2): 313-314.
- ↑ 김완재 (2009년 8월). 라디안의 속성에 관한 연구: 1rad은 각인가 실수인가?. 《수학교육학연구》 19 (3): 446.
- ↑ Hatice Akkoc (2008년 10월). Pre-service mathematics teachers’ concept images of radian. 《International Journal of Mathematical Education in Science and Technology》 39 (7): 876.
- ↑ Raymond A. Barnett, Michael R. Ziegler, Karl E. Byleen (2000년 7월 1일). 〈Chapter 5, Section 1〉, 《Precalculus: Functions and Graphs》, 5th edition, McGraw-Hill Science/Engineering/Math. ISBN 978-0072368710. 2016년 4월 12일에 확인.
- ↑ Raymond A. Barnett, Michael R. Ziegler, Karl E. Byleen (2000년 7월 1일). 〈Chapter 5, Section 2〉, 《Precalculus: Functions and Graphs》, 5th edition, McGraw-Hill Science/Engineering/Math. ISBN 978-0072368710. 2016년 4월 12일에 확인.