| 수포자도 쉽게 알 수 있는 수학 | ||||
|---|---|---|---|---|
| 집합과 명제 | 수의 체계와 수의 성질 | 문자와 식 및 방정식과 부등식 | 함수 | 수열 |
| 미분과 적분 | 확률과 통계 | 평면기하학과 공간기하학 | 해석기하학 | |
집합[편집 | 원본 편집]
일단 집합이 쉬운 개념은 아니라는 것부터 인식할 필요가 있다. 중학교 1학년 첫단원이라서 그런가, 다들 그다지 어렵게 생각 안해서 그렇지(고등 교육과정으로 넘어갔다) 집합이란 사실 대단히 추상적인 개념으로, 대단히 최근에(예를 들어 미적분보다 더 요즘) 생겨난 개념이다. 만일 이 단원이 어렵지 않게 느껴진다면 이미 수학적 사고와 추상화에 대단히 익숙해져 있기 때문이다. 거기에 익숙하지 않은 사람들은 당연히 이 단원이 어렵다!
집합은 결국 수학적 엄밀성과 연결되어 있다. 칸토어가 수학적 엄밀성을 추구하다보니 집합이란 개념이 생각난 것이고, 이는 1960년대의 새수학 운동과도 연결되어 있으므로, 이 단원을 언제 가르쳐야 할지 현재 애매한 상태이다. 현재는 수학 교과에서 가르친다.
간단히 말해서, 집합이란 건 모임이다. 우리 가족의 모임을 예로 들면 울아빠, 울엄마, 나, 우리동생 이렇게 표현할 수 있는 것처럼 이를 기호로 우리 가족={울아빠, 울엄마, 나, 우리동생} 이렇게 표현한 것이다. 그런데, 유의할 점이, 집합 안에도 원소로 집합이 들어갈 수 있다는 점이다. 예를 들면, 추석 때 모이는 친족 모임={우리 가족, 큰아버지 가족, 작은아버지 가족} 이런 방식으로 가족이라는 집합을 원소로 또 다른 집합을 만들 수 있는 것이다. 여기서 헷갈리지?
- 공집합: 원소가 하나도 없는 집합. 기호는 [math]\displaystyle{ \{ \} }[/math]나 [math]\displaystyle{ \emptyset }[/math].
[math]\displaystyle{ \phi }[/math]와는 다르다 [math]\displaystyle{ \phi }[/math]와는공집합은 모든 집합의 부분집합이다. - 전체집합: 고려해야하는 모든 대상을 담은 집합. 기호로는 보통 [math]\displaystyle{ U }[/math](universal set)를 쓴다.
집합 원소의 개수[편집 | 원본 편집]
A가 유한집합일 때, A의 원소의 개수를 [math]\displaystyle{ n(A) }[/math]로 표기한다. 나중에는 [math]\displaystyle{ |A| }[/math]의 표기도 쓴다.
집합의 연산[편집 | 원본 편집]
합집합[편집 | 원본 편집]
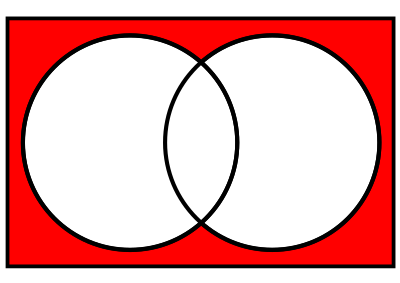
A에 속하거나 B에 속하는 모든 원소로 이루어진 집합을 A와 B의 합집합(union)이라고 하며 [math]\displaystyle{ A \cup B }[/math]로 표현한다.
여집합은 논리 연산자 OR([math]\displaystyle{ \lor }[/math])과 연관된다. 즉, [math]\displaystyle{ x \in A \cup B \iff x \in A \lor x \in B }[/math]이다.
교집합[편집 | 원본 편집]
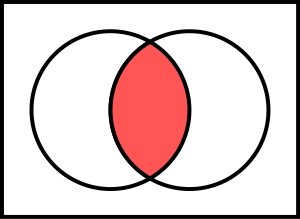
A와 B에 동시에 속하는 원소로 이루어진 집합을 A와 B의 교집합(intersection)이라고 하며 [math]\displaystyle{ A \cap B }[/math]로 표기한다.
교집합은 논리 연산자 AND([math]\displaystyle{ \land }[/math])와 연관된다. 즉, [math]\displaystyle{ x \in A \cap B \iff x \in A \land x \in B }[/math]이다.
[math]\displaystyle{ A \cap B = \emptyset }[/math]이면 A와 B는 서로 소(disjoint)라고 한다. 반대로 [math]\displaystyle{ A \cap B \neq \emptyset }[/math]이면 A와 B는 intersect한다고 하는데, 우리말로는 적당한 번역어가 없다.
여집합[편집 | 원본 편집]
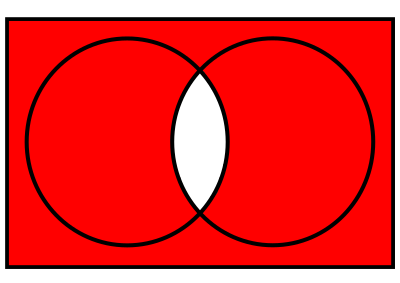
전체집합 U의 원소 중에서 A에 속하지 않는 모든 원소로 이루어진 집합을 (U에 대한) A의 여집합(complement)이라고 하고, [math]\displaystyle{ A^{c} }[/math]로 표기한다. 달리 표현하면 [math]\displaystyle{ A^{c} = U - A }[/math]이다.
여집합은 논리 연산자 NOT([math]\displaystyle{ \neg }[/math], 고등학교에서는 [math]\displaystyle{ \sim }[/math]를 더 많이 쓴다)과 연관된다. 즉, [math]\displaystyle{ x \in A^{c} \iff \neg[x \in A] }[/math]이다.
차집합[편집 | 원본 편집]
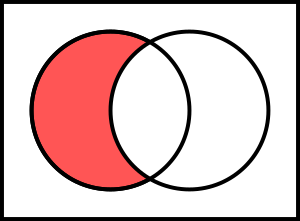
집합 A의 원소 중 집합 B의 원소가 아닌 것의 집합을 A에서 B를 뺀 차집합(difference)이라고 하며, [math]\displaystyle{ A-B }[/math]로 표기한다. 나중에는 [math]\displaystyle{ A \setminus B }[/math]의 표기도 쓴다.
[math]\displaystyle{ A-B = A \cap B^{c} }[/math]의 관계가 성립한다.
집합에 사용되는 정리, 법칙[편집 | 원본 편집]
포함-배제의 원리[편집 | 원본 편집]
합집합의 원소의 개수를 셀 때 사용하는 방법이다. 경우의 수를 셀 때 아주 유용하게 쓰인다. [math]\displaystyle{ n \left( A \cup B \right) = n \left( A \right) + n \left( B \right) - n \left( A \cap B \right) }[/math]
[math]\displaystyle{ n \left( A \cup B \cup C \right) = n \left( A \right) + n \left( B \right) + n \left( C \right) - n \left( A \cap B \right) - n \left( B \cap C \right) - n \left( C \cap A \right) + n \left( A \cap B \cap C \right) }[/math]
집합이 3개 이상일 때에도 비슷한 방법으로 계산할 수 있다.
드 모르간의 법칙[편집 | 원본 편집]
[math]\displaystyle{ \left( A \cup B \right) ^c = A^c \cap B^c }[/math]
[math]\displaystyle{ \left( A \cap B \right) ^c = A^c \cup B^c }[/math]
집합의 연산식을 단순하게 만들 때 사용한다.
[math]\displaystyle{ \left( A \cup B \right) ^c = A^c \cap B^c, \left( A \cap B \right) ^c = A^c \cup B^c }[/math]
만약 괄호 안에 둘 이상의 집합의 합집합 또는 교집합 연산이 포함된 식이 있을 때는 결합 법칙이 성립하므로 괄호 안을 두 개의 괄호로 나누면 된다.(?? 아래 식 잘못된것같음. 한 괄호 안에 합집합과 교집합이 공존할 순 없잖나...)
[math]\displaystyle{ \left( A \cup B^c \cap C \cap D^c \right) ^c }[/math]
[math]\displaystyle{ = \left( \left( A \cup B^c \right) \cap \left( C \cap D^c \right) \right) ^c }[/math]
[math]\displaystyle{ = \left( \left( A \cup B^c \cap C \right) \cap D^c \right) ^c }[/math]
[math]\displaystyle{ = \left( A \cup \left( B^c \cap C \cap D^c \right) \right) ^c }[/math]
명제[편집 | 원본 편집]
누구라도 참인지 거짓인지 일치된 판단을 할 수 있는 문장을 명제라고 한다. 즉, 객관적으로 참과 거짓을 알 수 있다.
명제의 구조[편집 | 원본 편집]
특정한 변수의 값에 따라 참과 거짓이 달라지는 식 또는 문장을 조건이라고 한다.
- 예시
- x는 정수이다.
- (어떤 도형이) 삼각형이면 내각의 합이 180도이다.
- 추가바람
조건은 명제를 이루는 가장 작은 요소이로, 명제는 조건 p, q를 이용해 "p이면 q이다"와 같이 만들어질 수 있다.
더이상 쪼갤 수 없는 가장 작은 단위의 명제를 단순명제라고 한다. 조건과는 다르다! 조건과는!
- 예시
- 위키러는 사람이다.
- 리브라는 실존인물이다.
그랬으면 좋겠..
위의 예시를 보면 공통적으로 "A는 B이다" 형식으로 되어 있는 것을 볼 수 있다. A에는 명제가 표현하고자 하는 대상이 오고, B에는 그 대상의 특성이 오게 된다.
단순 명제들 여러 개로 이루어진 하나의 명제를 합성명제라고 한다.
- 예시
마찬가지로 예시로 알 수 있듯이, A이면 B이다 형식으로 되어 있는 것을 볼 수 있다. A를 가정, B를 결론이라고 한다. 따라서, 복합명제는 "A라고 가정했을 때 B라는 결론이 나온다" 라는 뜻으로 해석할 수 있다.
진리표[편집 | 원본 편집]
명제의 모든 가능성을 표로 나열한 것을 진리표라고 한다.
단순명제 p의 진리표는 다음과 같이 작성한다.
| p |
|---|
| T |
| F |
(여기에서 T는 참, F는 거짓을 나타낸다)
합성명제 [math]\displaystyle{ p \rightarrow q }[/math]의 진리표는 다음과 같은 방식으로 작성한다.
| p | q | [math]\displaystyle{ p \rightarrow q }[/math] |
|---|---|---|
| T | T | T |
| T | F | F |
| F | T | T |
| F | F | T |
진리표를 그린 결과가 모두 참일 때, 그 명제를 항진명제라고 하고, 모두 거짓일 때는 모순이라고 한다.
논리 연산[편집 | 원본 편집]
단순명제를 논리 연산을 이용해 합성하면 합성명제를 만들어낼 수 있다. 이 문서에서 알아볼 논리 연산의 종류는 다음과 같다.
- [math]\displaystyle{ \lnot }[/math](not)
| p | [math]\displaystyle{ \lnot }[/math]p |
|---|---|
| T | F |
| F | T |
- [math]\displaystyle{ \land }[/math](and)
| p | q | p[math]\displaystyle{ \land }[/math]q |
|---|---|---|
| T | T | T |
| T | F | F |
| F | T | F |
| F | F | F |
- [math]\displaystyle{ \lor }[/math](or)
| p | q | p[math]\displaystyle{ \lor }[/math]q |
|---|---|---|
| T | T | T |
| T | F | T |
| F | T | T |
| F | F | F |
- [math]\displaystyle{ \rightarrow }[/math]
p[math]\displaystyle{ \rightarrow }[/math]q는 [math]\displaystyle{ \lnot }[/math]p[math]\displaystyle{ \lor }[/math]q와 같다.
p[math]\displaystyle{ \rightarrow }[/math]q가 참일 때 p[math]\displaystyle{ \Rightarrow }[/math]라고 쓴다.
| p | q | p[math]\displaystyle{ \rightarrow }[/math]q |
|---|---|---|
| T | T | T |
| T | F | F |
| F | T | T |
| F | F | T |
- [math]\displaystyle{ \leftrightarrow }[/math]
p[math]\displaystyle{ \leftrightarrow }[/math]q는 (p[math]\displaystyle{ \rightarrow }[/math]q)[math]\displaystyle{ \land }[/math](q[math]\displaystyle{ \rightarrow }[/math]p) 와 같다.
p[math]\displaystyle{ \leftrightarrow }[/math]q가 참일 때 p[math]\displaystyle{ \Leftrightarrow }[/math]라고 쓴다.
| p | q | p[math]\displaystyle{ \leftrightarrow }[/math]q |
|---|---|---|
| T | T | T |
| T | F | F |
| F | T | F |
| F | F | T |
연산법칙[편집 | 원본 편집]
모든 논리연산은 [math]\displaystyle{ A\land B, A\lor B, \lnot A }[/math](A, B는 임의의 논리식)만으로 표현이 가능하다. 이것에 대한 자세한 설명은 논리연산참고. 이 연산들에 대해 다음 법칙이 성립한다.
- 교환법칙, 결합법칙, 분배법칙
- 흡수법칙
- A[math]\displaystyle{ \land }[/math](A[math]\displaystyle{ \lor }[/math]B)=A
- A[math]\displaystyle{ \lor }[/math](A[math]\displaystyle{ \land }[/math]B)=A
- 드모르간의 법칙
- ([math]\displaystyle{ \lnot }[/math]A)[math]\displaystyle{ \land }[/math]([math]\displaystyle{ \lnot }[/math]B)=[math]\displaystyle{ \lnot }[/math](A[math]\displaystyle{ \lor }[/math]A)
- ([math]\displaystyle{ \lnot }[/math]A)[math]\displaystyle{ \lor }[/math]([math]\displaystyle{ \lnot }[/math]B)=[math]\displaystyle{ \lnot }[/math](A[math]\displaystyle{ \land }[/math]A)
- 기타
- A[math]\displaystyle{ \land }[/math]A=A
- A[math]\displaystyle{ \lor }[/math]A=A
- A[math]\displaystyle{ \land }[/math]0=0
- A[math]\displaystyle{ \lor }[/math]0=A
- A[math]\displaystyle{ \land }[/math]1=A
- A[math]\displaystyle{ \lor }[/math]1=1
- A[math]\displaystyle{ \land }[/math]([math]\displaystyle{ \lnot }[/math]A)=0
- A[math]\displaystyle{ \lor }[/math]([math]\displaystyle{ \lnot }[/math]A)=1
- [math]\displaystyle{ \lnot }[/math]([math]\displaystyle{ \lnot }[/math]A)=A
각각의 연산법칙들은 밴 다이어그램을 그려보면 쉽게 이해할 수 있다.
역, 이, 대우[편집 | 원본 편집]
명제 [math]\displaystyle{ p\rightarrow q }[/math]는 세 가지 방법으로 뒤집을 수 있다.
- 가정과 결론을 바꾸는 방법([math]\displaystyle{ q\rightarrow p }[/math])을 역이라고 한다.
- 가정과 결론을 모두 부정하는 방법([math]\displaystyle{ \lnot p\rightarrow\lnot q }[/math])을 이라고 한다.
- 역과 이를 동시에 적용하는 방법([math]\displaystyle{ \lnot q\rightarrow\lnot p }[/math])을 대우라고 한다.
이들의 특성은 다음과 같다.
- 원명제와 역명제, 이명제와 대우명제는 서로 역 관계에 있다.
- 원명제와 이명제, 역명제와 대우명제는 서로 이 관계에 있다.
- 원명제와 대우명제, 역명제와 이명제는 서로 대우 관계에 있다.
공리[편집 | 원본 편집]
우리가 어떤 명제의 참과 거짓을 증명해낼 때는 다른 명제를 이용하게 된다. 그런데, 모든 명제가 처음부터 참과 거짓이 증명되어 있지 않다면? 충격과 공포다 전제가 이상하기 때문에 증명이 맞다고 할 수 없게 된다. 올바른 증명을 하려면 가장 기초적인 근거가 될 가정을 할 필요가 있다. 이것을 공리라고 한다. 어떤 이론체계에서든 공리를 제대로 설정했을 때만 논리적으로 접근할 수 있다.
공리로 시작해서 연역적으로 유도되는 명제를 정리라고 한다.
집합과의 관계[편집 | 원본 편집]
변수가 속해있는 전체집합 U의 원소 중 어떤 조건이 참이 되게 하는 모든 원소의 집합을 그 조건의 진리집합이라고 한다. 조건 p, q의 진리집합이 P, Q일 때, 다음이 성립한다.
(p[math]\displaystyle{ \Rightarrow }[/math]q) [math]\displaystyle{ \Leftrightarrow }[/math] (P[math]\displaystyle{ \in }[/math]Q)
(p[math]\displaystyle{ \nRightarrow }[/math]q) [math]\displaystyle{ \Leftrightarrow }[/math] (P[math]\displaystyle{ \notin }[/math]Q)
각주
| 쉽게 알 수 있는 학문 | |
|---|---|
| 쉽게 할 수 있는 취미 | |
| 창작 안내서 시리즈 | |
| 뭘헤매지 시리즈 | |
| 여행 시리즈 | |
| 연표 시리즈 | |
| 생활의 지혜 | |
| 리브레 기네스 | |
| 리브레 위키 | |
| 기타 | |