변심거리(邊心距離,apothem 또는 줄여서 apo)또는 '정다각형의 변심거리'는 기하학에서 정다각형의 중심에서 변까지의 거리를 말한다.
또는 원의 중심을 지나는 직선이 현을 수직이등분할때 중심부터 현까지의 거리를 말한다.
정사각형[편집 | 원본 편집]
원에 내접한 정다각형의 변심거리(apo)는 연장선상의 화살거리(sagit)와 더해져 반지름이 된다.
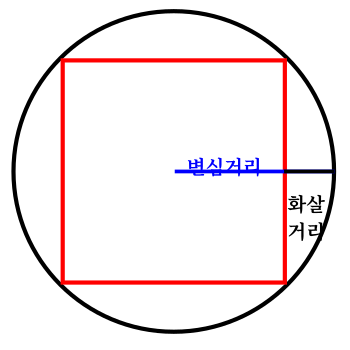
|
| 원에 내접한 정사각형의 한변에 도달한 변심거리와 원의 반지름 그리고 그 둘의 간격 차인 화살거리 |
현[편집 | 원본 편집]
변심거리의 변을 현으로 가정해볼때 현을 수직이등분하는 직선이 원의 중심을 지나는것과 관련있음을 확인할수있다. [1][2] 또한 직경과 현의 길이와의 관계는 피타고라스의 정리를 통해서 조사할수있다.
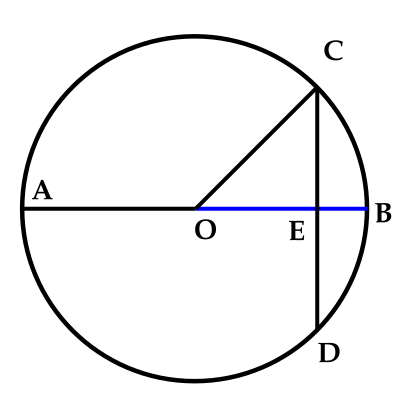
|
| 유클리드 기하학 원론 제3권 법칙35 |
현 [math]\displaystyle{ \overline{CD} }[/math]와 직경[math]\displaystyle{ \overline{AB} }[/math]가 수직으로 만나는 점 E에서 선분 AE와 선분EB와의 비율은 원둘레에서 호의 길이의 비율을 보여준다. 따라서 이러한 성질은 선분 AE와 선분EB와의 비율에서 역시 활꼴의 면적을 보여줄 수 있다.
- [math]\displaystyle{ \overline{AB} }[/math]가 원의 중심을 지나 현 [math]\displaystyle{ \overline{CD} }[/math]를 수직이등분할때
- [math]\displaystyle{ \overline{OC}^2 = \overline{CE}^2+ \overline{OE}^2 }[/math]
- [math]\displaystyle{ \overline{OC} = \overline{OB} = \overline{AO} }[/math]
- [math]\displaystyle{ \overline{OB}= \overline{OE} + \overline{EB} }[/math]
따라서
- [math]\displaystyle{ \overline{CE}^2 =\overline{OC}^2 - \overline{OE}^2 }[/math]
- [math]\displaystyle{ \overline{CE}^2 =\overline{AO}^2 - \overline{OE}^2 }[/math]
그리고
- [math]\displaystyle{ \overline{AO}^2 - \overline{OE}^2 = \left( \overline{AO} -\overline{OE}\right) \left(\overline{AO}+ \overline{OE} \right) }[/math]
- [math]\displaystyle{ \overline{AO}^2 - \overline{OE}^2 = \left( \overline{EB}\right) \left(\overline{AE} \right) }[/math]
따라서
- [math]\displaystyle{ \left( \overline{CE}\right) \left(\overline{CE} \right) =\left( \overline{EB}\right) \left(\overline{AE} \right) }[/math]
- [math]\displaystyle{ \left( \overline{CE}\right) \left(\overline{ED} \right) =\left( \overline{EB}\right) \left(\overline{AE} \right) }[/math]이다.
이렇게 현의 길이와 직경(지름)의 길이는 일대일 대응한다.
한편 활꼴의 길이 선분CD와 활꼴의 높이 선분EB를 갖는 활꼴의 길이([math]\displaystyle{ l }[/math])는 높이([math]\displaystyle{ h }[/math])와 직경([math]\displaystyle{ D }[/math])에서 다음의 관계가 있다.
- [math]\displaystyle{ \left( \overline{CE} \right) \left( \overline{ED} \right) = \left( \overline{AE} \right) \left( \overline{EB} \right) }[/math]이다.
- [math]\displaystyle{ \overline{CD} = \overline{CE} + \overline{ED} }[/math]이고 [math]\displaystyle{ \overline{CE} = \overline{ED} }[/math]이므로
- [math]\displaystyle{ \overline{AE} = a, \overline{EB} = b , \overline{CE} =x }[/math]를 가정하고
- [math]\displaystyle{ x^2 = ab }[/math]
- [math]\displaystyle{ x = \sqrt{ab} }[/math]
- [math]\displaystyle{ l = 2x = 2\sqrt{ab} }[/math]이고 [math]\displaystyle{ a =D-b }[/math]이다.
따라서
- [math]\displaystyle{ l = 2\sqrt{(D-b)b} }[/math]
따라서
- [math]\displaystyle{ l = 2\sqrt{(D-b)b} }[/math]
- [math]\displaystyle{ l = \sqrt{4} \sqrt{(D-h)h} }[/math]
- [math]\displaystyle{ l = \sqrt{ \left( 4D h \right) - \left( 4 h^2 \right) } }[/math]
연관[편집 | 원본 편집]
- [참고](유클리드 기하학 원론,구텐베르크 프로젝트,John Casey 1885)http://www.gutenberg.org/files/21076/21076-pdf.pdf?session_id=9bfd9ef535a37ac859a6028f101fa4451e3226cc
- ↑ [참고] (수학방 -원의 방정식,원의 방정식 표준형) https://mathbang.net/454
- ↑ 우리말샘 원 등